Tangential
$2,400.00
Tangential. Edition of 30. 2017.
Box size: 12-1/2 x 9-1/2 x 1″
Book size (closed): 12″ x 9″
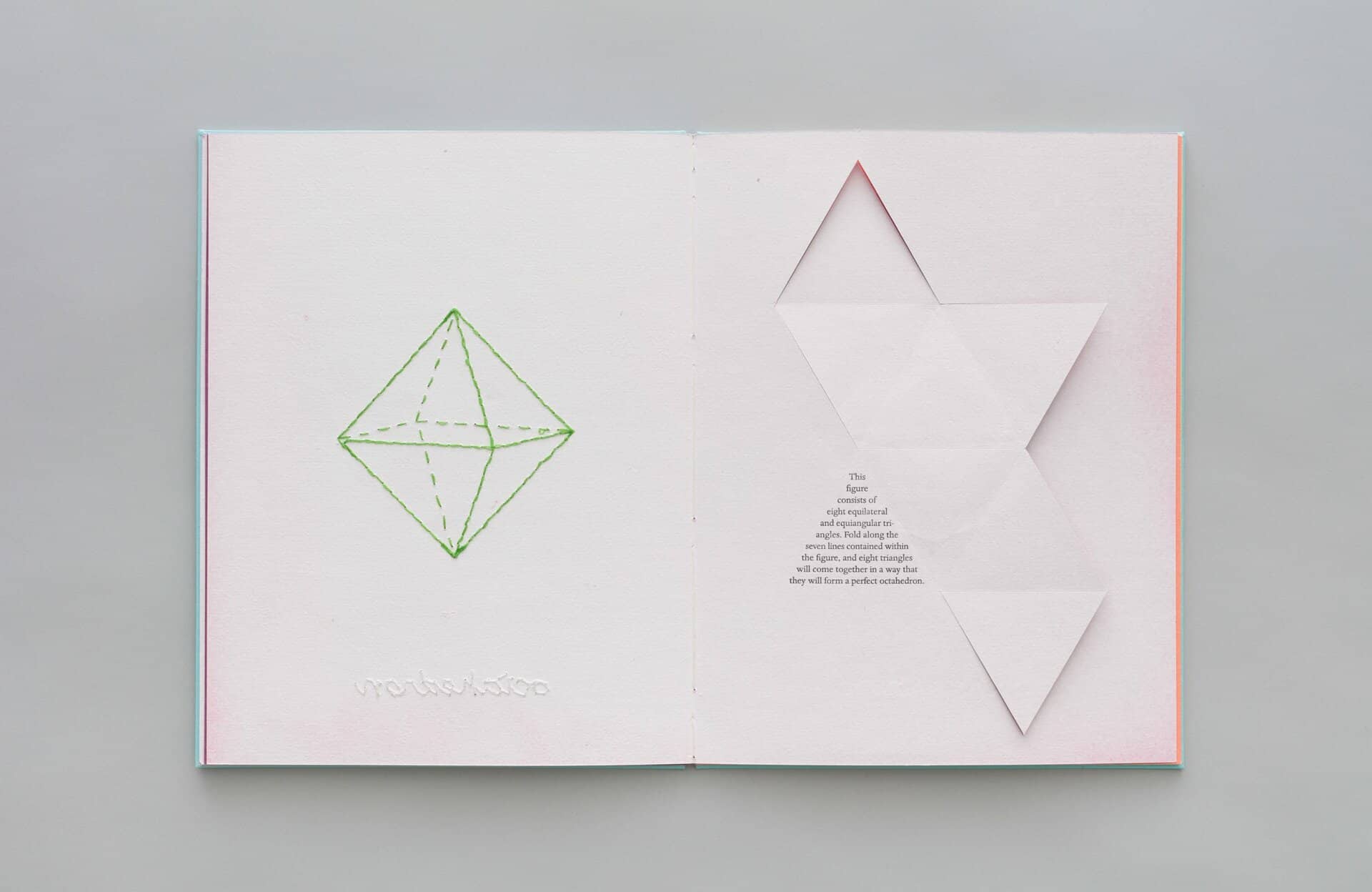
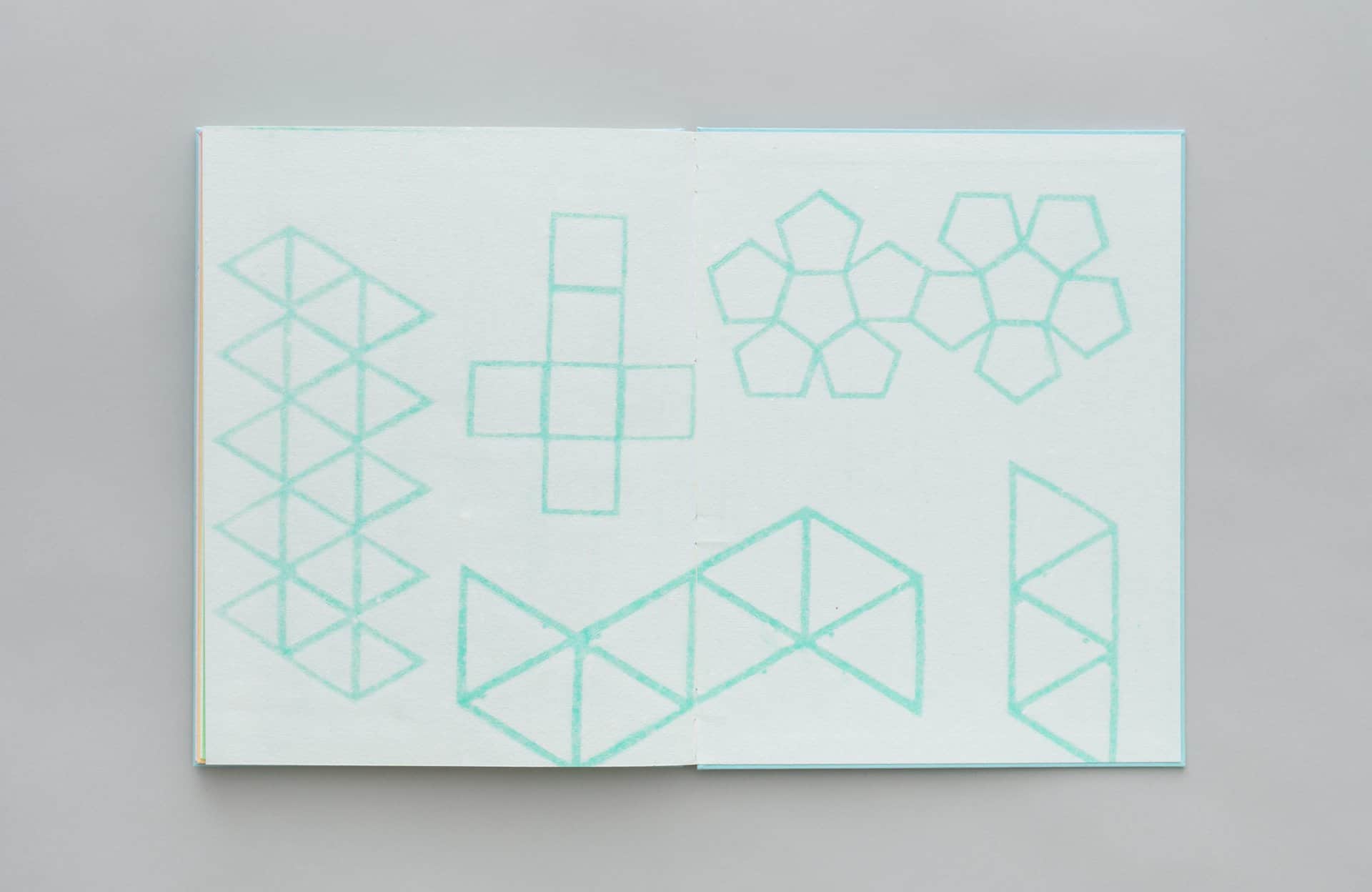
After viewing (and handling) the 1570 edition of Euclid’s Elements of Geometry at the Denver Public Library, I was drawn to Chapter 11, which focuses on polyhedra. I find it interesting to imagine how Euclid constructed his ideas in the physical world, since paper was invented later. Perhaps he constructed them in papyrus or parchment (precursors to paper). I am intrigued by the many ways in which a flat sheet of paper can transform from two- into three-dimensions. I was drawn to the tiny flaps glued into the pages of the old copy of Euclid’s Elements, which allow the reader to explore the physical relationships between lines, angles and surfaces.
Out of stock
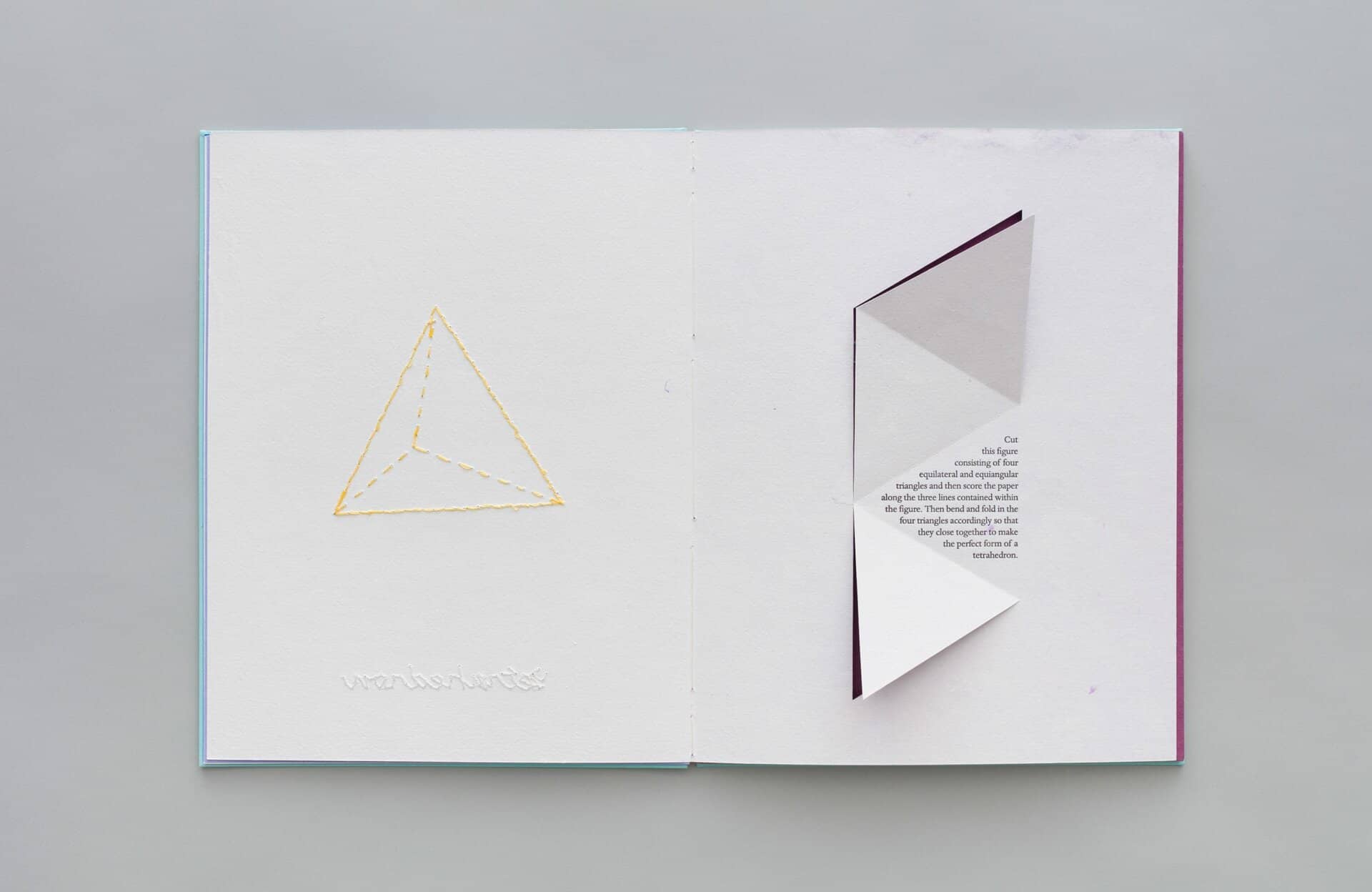
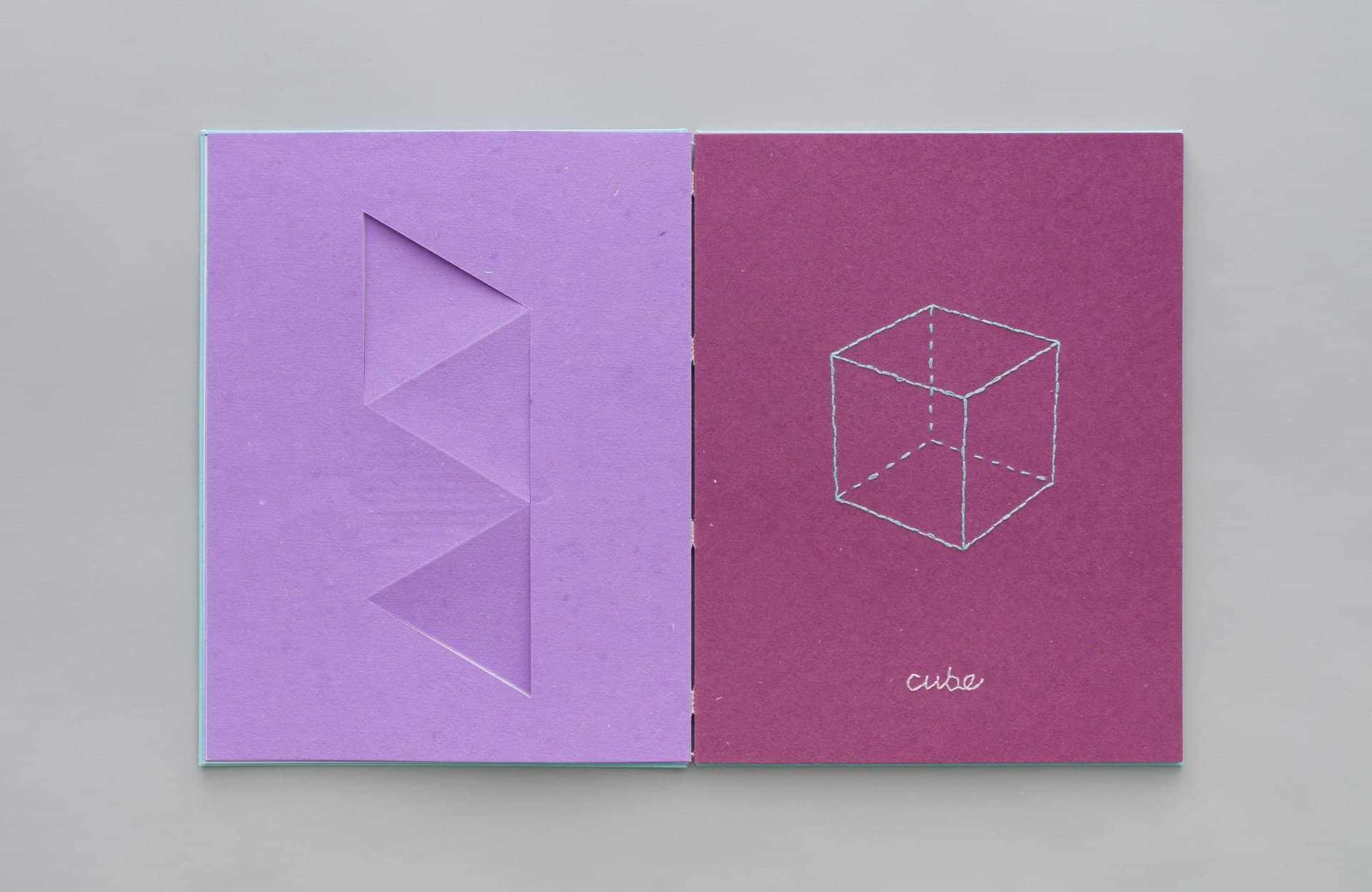
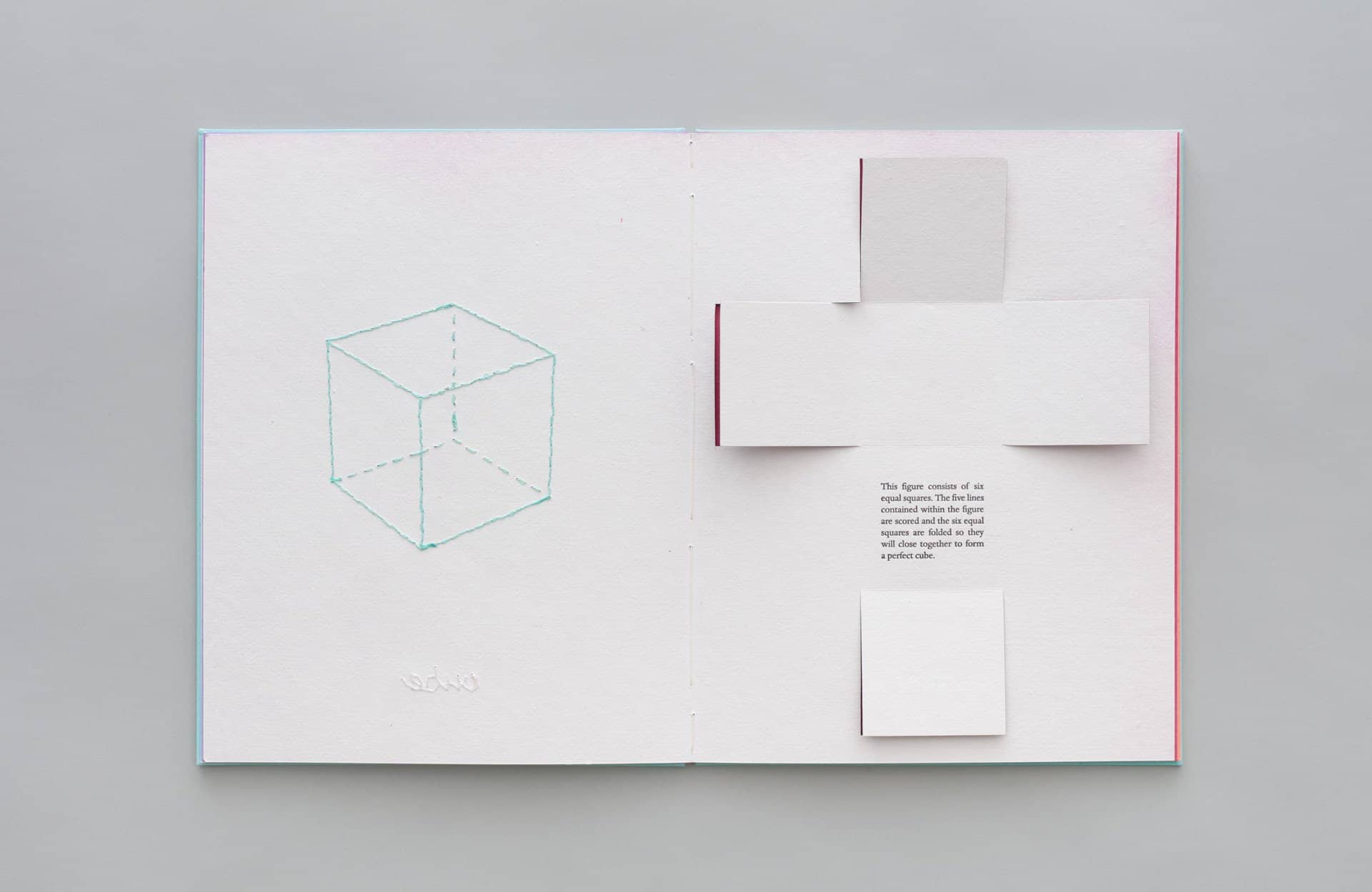
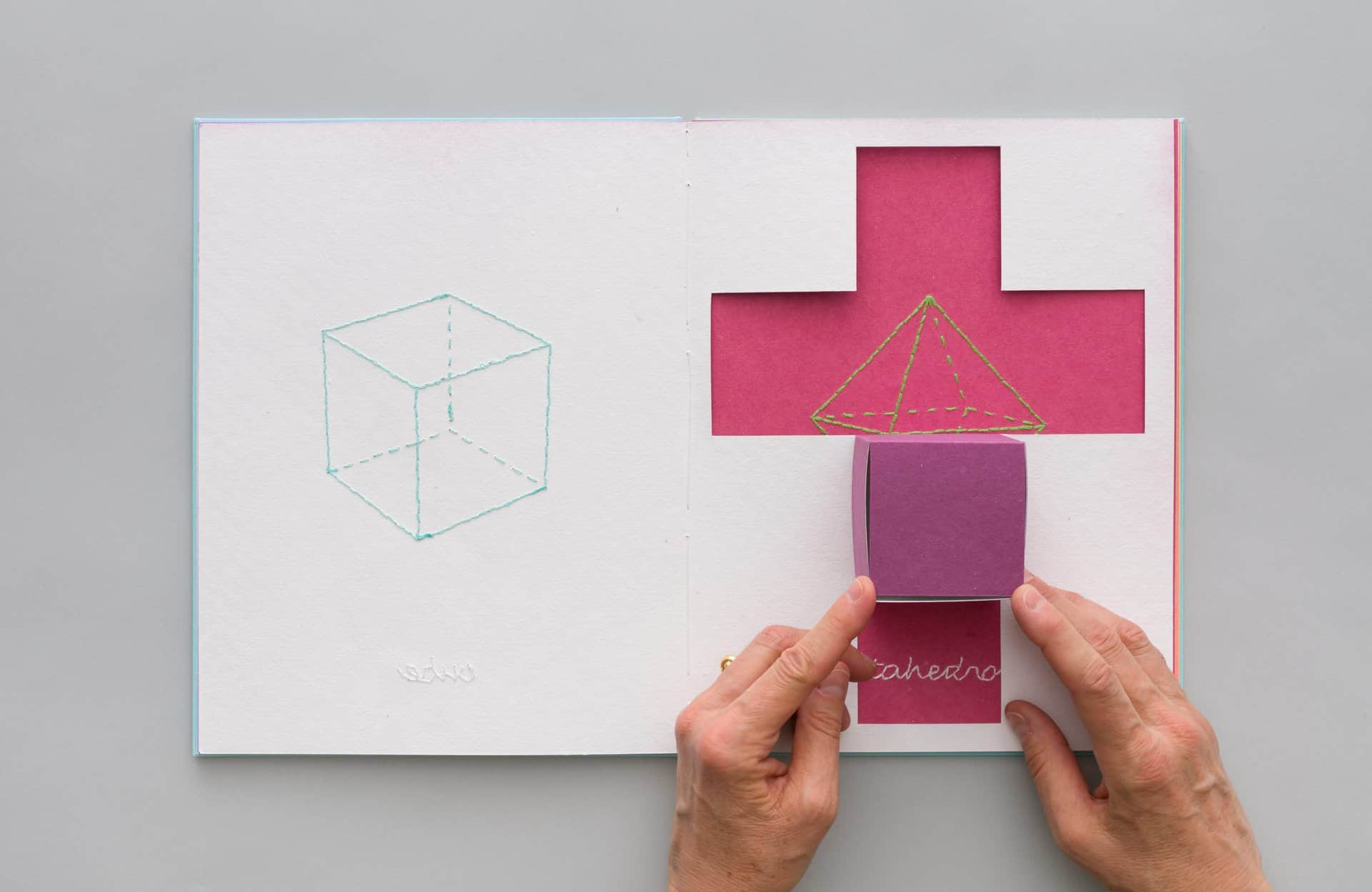
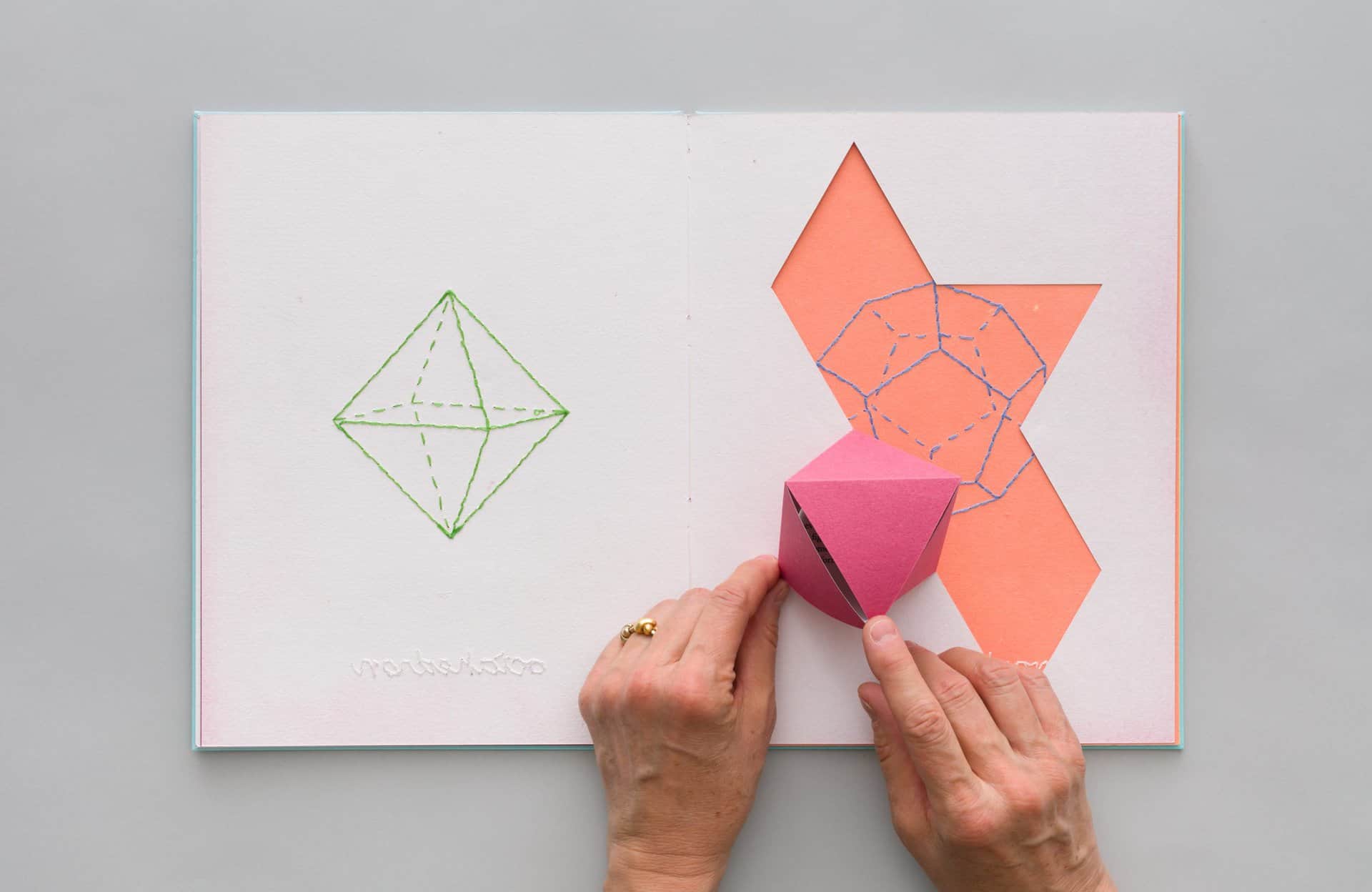
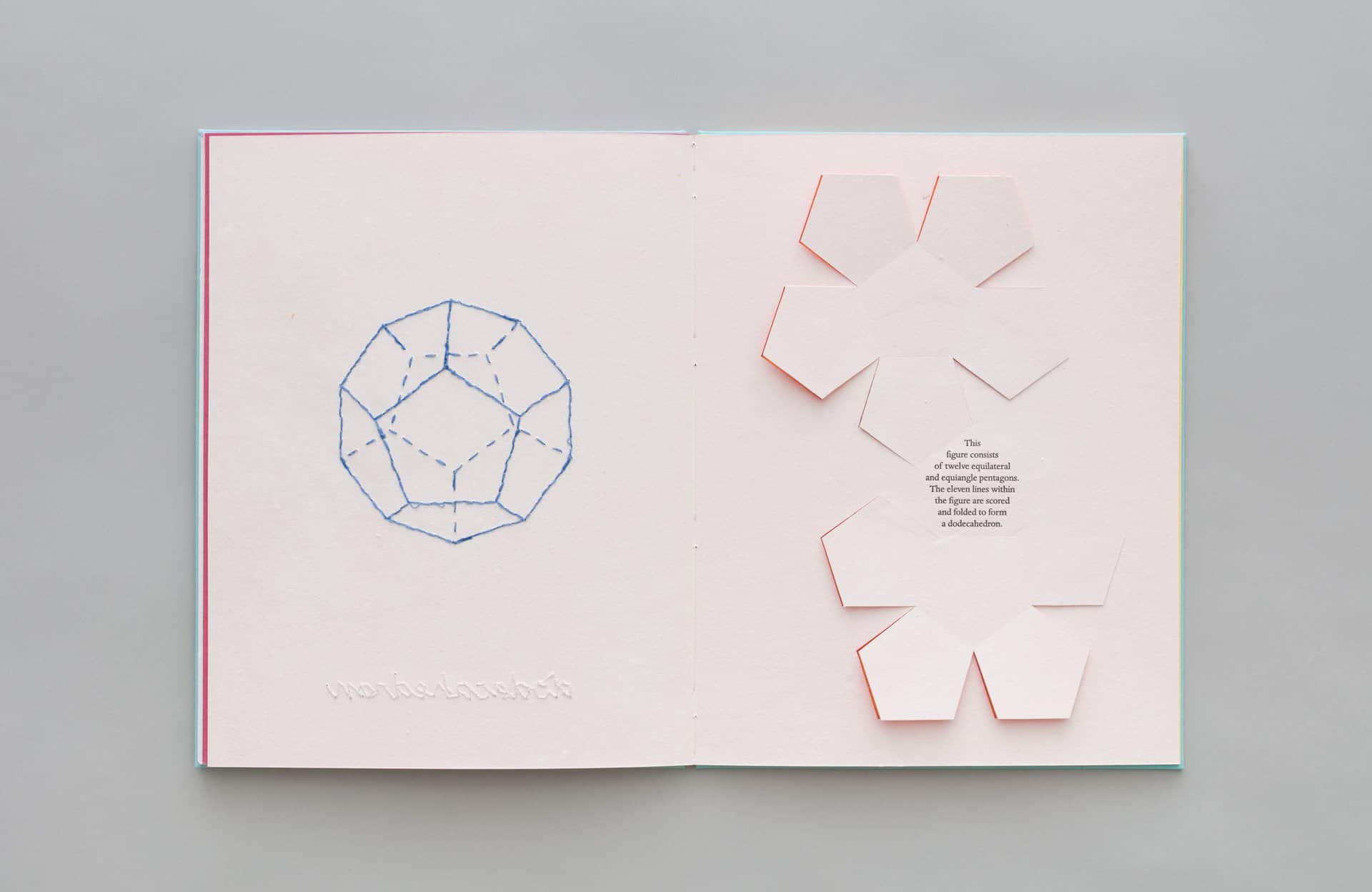
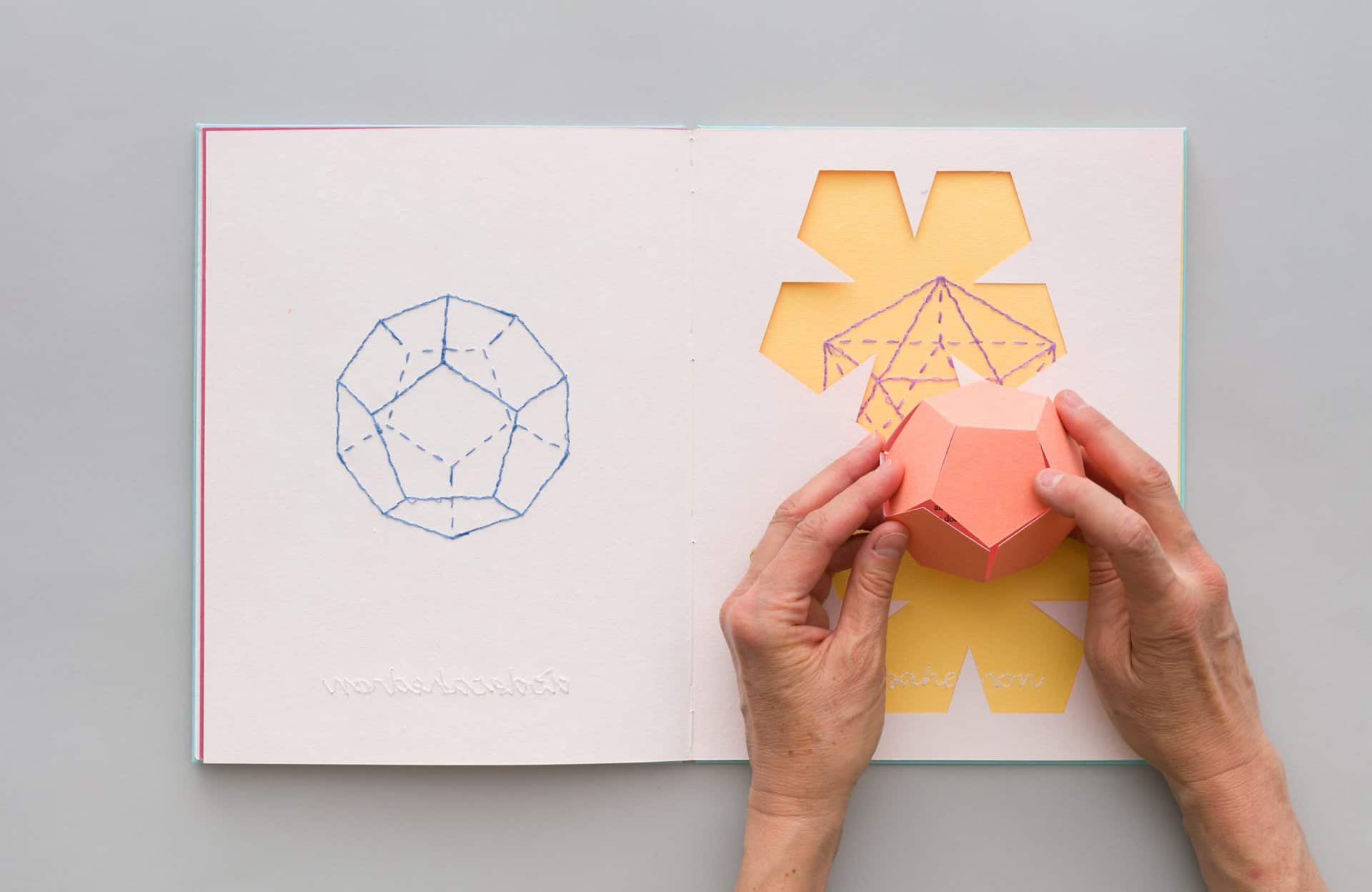
I built the basic polyhedra, or platonic solids, into the pages of the book (which are double-sided cotton handmade sheets). Each form lies flat on the page (like an architectural floor plan) with visible cut and score lines. The shapes can be lifted from the page (but not removed) and folded to create each polyhedra. The text is adapted from Euclid’s Elements (which was originally translated into old English) and is direct, simple and didactic.
The basic text is letterpress printed, and I hand stitched the name and a flat rendering of each three-dimensional shape (tetrahedraon, cube, octahedron, dodecahedron, icosahedron). I enjoy repetitive handwork and the interaction with the sheets as I stitch. I spend about an hour with each page, turning it back and forth as I insert the needle time and again.
Each folio is double sided. The color sequence (most visible on the spine) flows through the ten chromatic color fields, appearing sequentially. The geometric shapes begin flat and white, but when folded into their solid forms, they appear as colored shapes. As the pages turn, the shapes become more complex, beginning with four triangles that fold up into a tetrahedron and ending with a 20-sided icosahedron. The shapes in this book are meant to be formed on the page and then unfolded, allowing a mental image of each polyhedra to live in the mind beyond the physical experience.